Rotational Equilibrium

Rotational Equilibrium

When the net force on an object is zero we say that it is in translational equilibrium and its center of mass has zero acceleration. When the net torque on an object is zero we say that it is in rotational equilibrium and it has zero angular acceleration. This means that the torque trying to spin an object in one direction equals the torque trying to spin it in the opposite direction. We will use counterclockwise as a positive torque and clockwise as a negative torque. So τ_ccw = τ_cw when an object is in rotational equilibrium.

Rotational Equilibrium Problem Solving

1. Draw the object, including vectors (arrows) for all the forces acting on the object. The weight of the object itself can always be drawn at its center of mass.
2. Identify the pivot point where the object can rotate around. (If the object is not rotating you can choose any point that is convenient)
3. Measure the angle and distance from the pivot to each force.
4. Add up all the torques in the clockwise direction and set them equal to the torques in the counter clockwise direction.
5. Solve the equation

Sample Problem

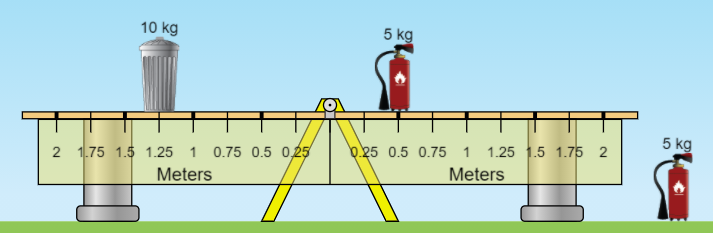
A 10kg garbage can is placed 1.25m from the central pivot on a teeter-totter as shown above. A 5kg fire extinguisher is placed .5m on the opposite side of the central pivot. Where should a second 5kg fire extinguisher be placed to balance the teeter-totter? You can solve this on your own and check your answer with the simulation below, or watch this video to see a solution.

Play with the simulation to help understand Rotational Equilibrium.

Writing equations for torque and net force.

The picture to the left shows a sign hanging from a uniformly dense beam that has mass \(M_b\). The sign has a mass of \(M_s\). The length of the beam is \(L\). A cable with tension \(T\) is attached to the end of the beam and makes an angle \(\theta\) with the beam. The hinge on the left side of the beam applies a horizontal force \(F_{Hx}\) and a vertical force up of \(F_{Hy}\). Write but do not solve equations for\(\sum F_x,\sum F_y, and \sum \tau \) in terms of the given variables and universal constants.